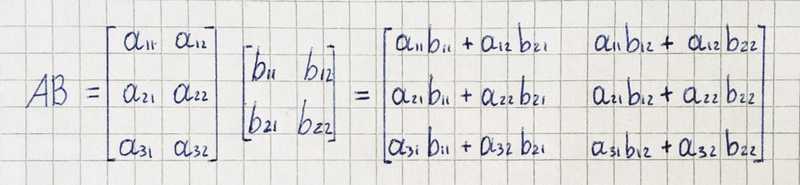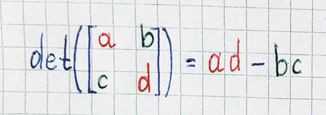Basic Matrix Operations With JavaScript

In this part, we will cover the most of basic matrix operations. We are going add and subtract matrices, multiply them by a scalar, implement matrix-matrix multiplication, find transpose matrix and take a more deep look at determinant. We are not going to look at concepts such as inverse matrix and rank in this part, but leave them for the future.

Addition and Subtraction

The matrix addition and subtraction operations take pairs of matrices as inputs and produce matrices as outputs. Addition and subtraction are performed component-wise and matrices must have the same dimensions to be added or subtracted.

To not repeat the same code in addition and subtraction we first write a method that receives operation, function that will do something with two components and another matrix. Then we can call it in both addition and subtraction methods with different operations.

class Matrix {
  // ...
  componentWiseOperation(func, { rows }) {
    const newRows = rows.map((row, i) =>
      row.map((element, j) => func(this.rows[i][j], element))
    )
    return new Matrix(...newRows)
  }
  add(other) {
    return this.componentWiseOperation((a, b) => a + b, other)
  }
  subtract(other) {
    return this.componentWiseOperation((a, b) => a - b, other)
  }
}

const one = new Matrix(
  [1, 2],
  [3, 4]
)
const other = new Matrix(
  [5, 6],
  [7, 8]
)

console.log(one.add(other))
// Matrix { rows: [ [ 6, 8 ], [ 10, 12 ] ] }
console.log(other.subtract(one))
// Matrix { rows: [ [ 4, 4 ], [ 4, 4 ] ] }

Scalar-Matrix Multiplication

Scalar multiplication of matrices is defined in a similar way as for vectors and is done by multiplying every element of the matrix by the scalar.

class Matrix {
  // ...
  scaleBy(number) {
    const newRows = this.rows.map(row =>
      row.map(element => element * number)
    )
    return new Matrix(...newRows)
  }
}

const matrix = new Matrix(
  [2, 3],
  [4, 5]
)
console.log(matrix.scaleBy(2))
// Matrix { rows: [ [ 4, 6 ], [ 8, 10 ] ] }

Matrix-Matrix Multiplication

We can multiply two matrices A and B provided their dimensions are compatible, which means the number of columns of A equals the number of rows of B. The matrix product AB of matrices consists of computing the dot product between each row of A and each column of B:

class Matrix {
  // ...
  multiply(other) {
    if (this.rows[0].length !== other.rows.length) {
      throw new Error('The number of columns of this matrix is not equal to the number of rows of the given matrix.')
    }
    const columns = other.columns()
    const newRows = this.rows.map(row =>
      columns.map(column => sum(row.map((element, i) => element * column[i])))
    )

    return new Matrix(...newRows)
  }
}

const one = new Matrix(
  [3, -4],
  [0, -3],
  [6, -2],
  [-1, 1]
)
const other = new Matrix(
  [3,  2, -4],
  [4, -3,  5]
)
console.log(one.multiply(other))
// Matrix {
//   rows:
//    [ [ -7, 18, -32 ],
//      [ -12, 9, -15 ],
//      [ 10, 18, -34 ],
//      [ 1, -5, 9 ] ]}

We can think of a result of a matrix-matrix product AB as a linear transformation that first applies linear transformation B and then A. To better understand this concept let’s go to the linear-algebra-demo project.

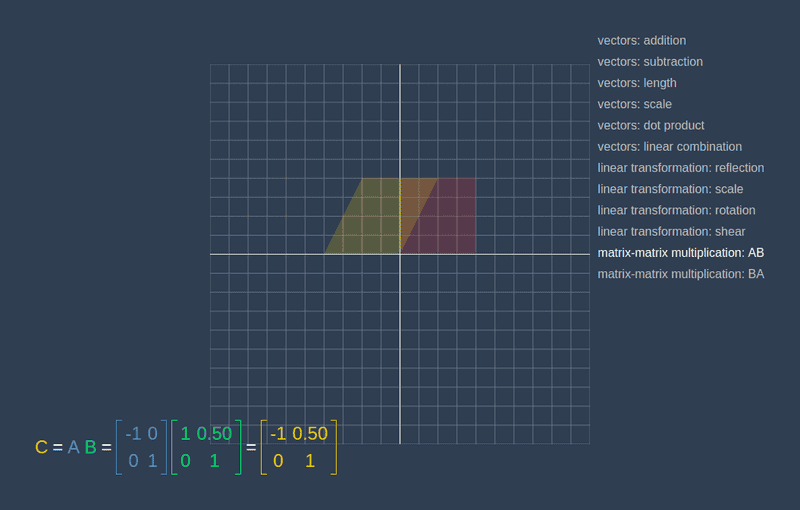
Yellow shape in the picture below is the result of applying linear transformation C to the red square. Transformation C is a result of matrix-matrix multiplication. Where A is a reflection matrix, and B is a shear matrix.

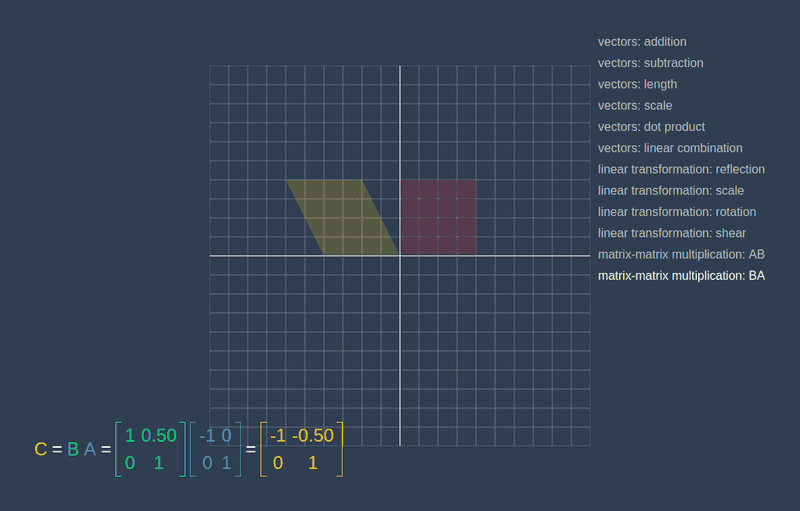
If we change the order of matrices A and B in matrix-matrix multiplication, we will receive a different result, because first will be applied transformation B and then A.

Transpose

The transpose matrix Aᵀ is defined by the formula aᵀᵢⱼ = aⱼᵢ. In other words, we obtain the transpose by “flipping” the matrix through its diagonal. Note that entries on the diagonal of the matrix are not affected by the transpose operation.

class Matrix {
  // ...
  transpose() {
    return new Matrix(...this.columns())
  }
}

const matrix = new Matrix(
  [0,  1,  2],
  [3,  4,  5],
  [6,  7,  8],
  [9, 10, 11]
)
console.log(matrix.transpose())
// Matrix {
//   rows: [
//     [ 0, 3, 6, 9 ],
//     [ 1, 4, 7, 10 ],
//     [ 2, 5, 8, 11 ]
//   ]
// }

Determinant

The determinant of a matrix is a calculation that involves all the coefficients of the matrix, and whose output is a single number. The determinant describes the relative geometry of the vectors that make up the rows of the matrix. More specifically, the determinant of a matrix A tells you the volume of a box with sides given by rows of A. The determinant of a 2×2 matrix is:

The determinant of a 3×3 matrix is:

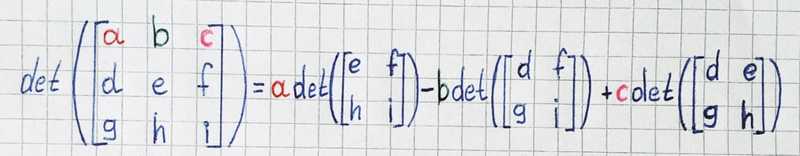
Our method will calculate determinant for a matrix of any size, as long as it has the same number of rows and columns.

class Matrix {
  // ...
  determinant() {
    if (this.rows.length !== this.rows[0].length) {
      throw new Error('Only matrices with the same number of rows and columns are supported.')
    }
    if (this.rows.length === 2) {
      return this.rows[0][0] * this.rows[1][1] - this.rows[0][1] * this.rows[1][0]
    }

    const parts = this.rows[0].map((coef, index) => {
      const matrixRows = this.rows.slice(1).map(row => [ ...row.slice(0, index), ...row.slice(index + 1)])
      const matrix = new Matrix(...matrixRows)
      const result = coef * matrix.determinant()
      return index % 2 === 0 ? result : -result
    })

    return sum(parts)
  }
}

const matrix2 = new Matrix(
  [ 0, 3],
  [-2, 1]
)
console.log(matrix2.determinant())
// 6
const matrix3 = new Matrix(
  [2, -3,  1],
  [2,  0, -1],
  [1,  4,  5]
)
console.log(matrix3.determinant())
// 49
const matrix4 = new Matrix(
  [3, 0, 2, -1],
  [1, 2, 0, -2],
  [4, 0, 6, -3],
  [5, 0, 2,  0]
)
console.log(matrix4.determinant())
// 20

Determinant shows us exactly how much are things being stretched. We can think of it as the factor by which a linear transformation changes any area. To better understand this concept let’s go to the linear-algebra-demo project.

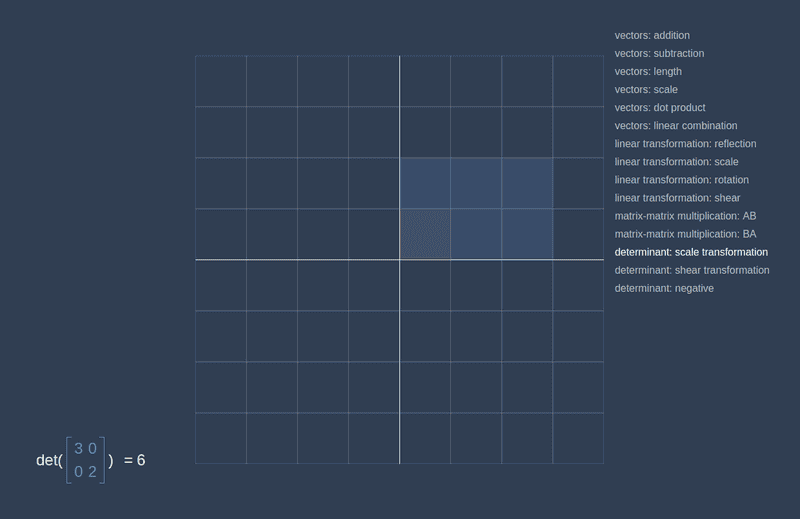
In the picture below we can see that after applying the linear transformation area to red 1×1 square we get a 3×2 rectangle with area equal to 6, exactly the same number as a determinant of the matrix.

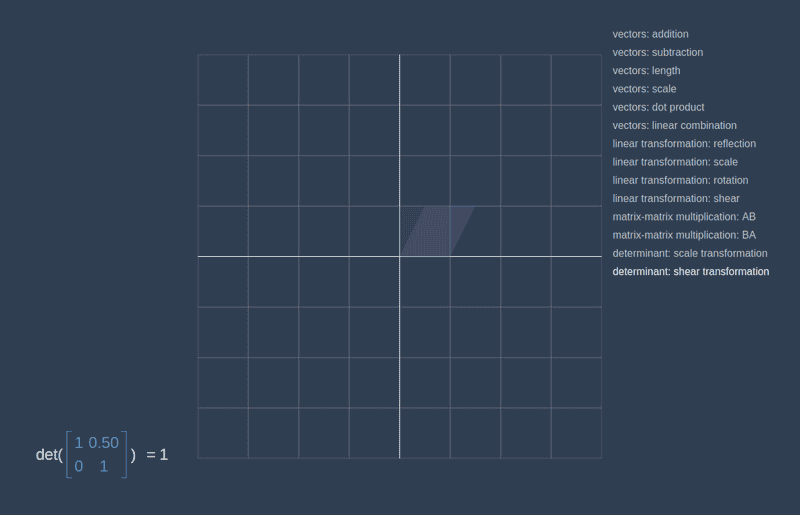
If we apply a shear transformation, we can see that the square become parallelogram, and the area remains the same. A determinant is equal to one.

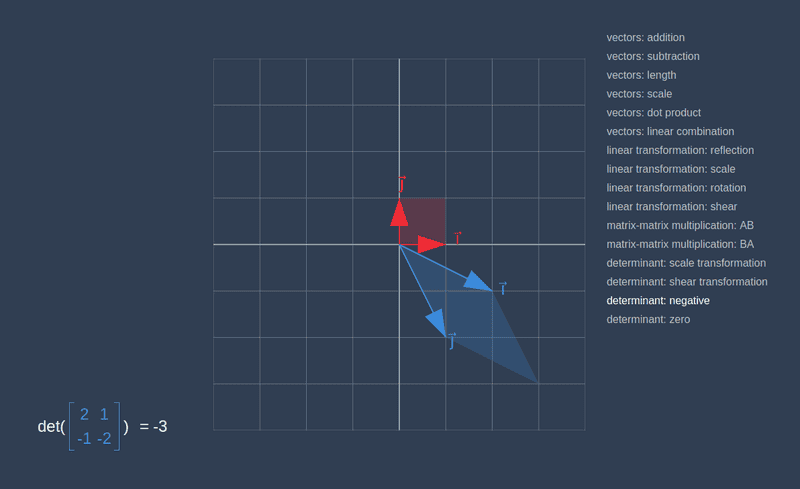
The determinant is negative if after applying the linear transformation orientation of the space will be reverted. In the picture below we can see that before transformation ĵ is to the left of î, and after the transformation ĵ on the right of î.

The determinant of a transformation is zero if it squishes all of space onto a line or even on a single point. It means checking if the determinant of a given matrix is 0 will give away if computing weather or not the transformation associated with that matrix squishes everything into a smaller dimension.

In three-dimensional space, determinant tells you how much volumes get scaled.

The determinant of 0 would mean that all of space is squished onto something with 0 volume. If in two-dimensional space it would mean that result of transformation squished onto a line or point, in three-dimensional case it also can mean that objects squished onto a plane, as in the example below.